from recent CERN PRESS RELEASE:
"The Standard Model Higgs mechanism is one of a range of ways that
fundamental particles could acquire their masses. According to the Higgs
mechanism, space is filled with a so-called Higgs field with which
particles interact. Those that interact strongly with the field have more
mass than those that interact weakly, rather like a streamlined racing car
cuts through air more easily than a bus."
This is for the fundamental leptons and quarks
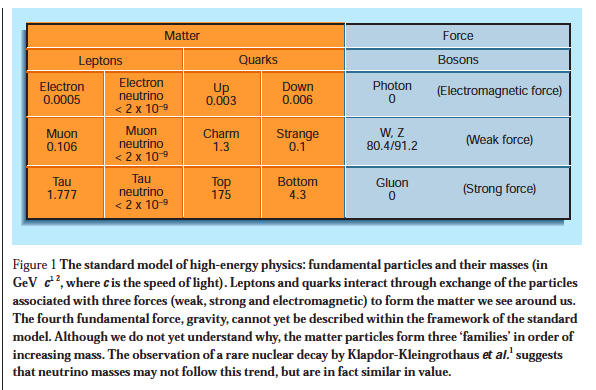
diagram by Ed Witten
On Aug 22, 2011, at 8:26 AM, Paul Zielinski wrote:
I have to agree with Jack that the Higgs mechanism already represents an anti-Machian solution to the problem of the origin of inertia. As I understand it, the Higgs model involves local interaction between matter in forced motion, and the physical vacuum.
Right. However, if one invokes the hologram model then the Machian idea reappears at a deeper level. But one needs retro-causal Wheeler-Feynman ideas on steroids to really get it to work in the sense of a grand Novikov self-consistent time loop in a "block universe" picture. Very speculative of course. Crazy idea - crazy enough to be true?
.jpeg)
adapted from Tamara Davis Ph.D.
On 8/21/2011 2:02 PM, Jack Sarfatti wrote:
Gravity is the pattern of geodesics.
>
> The inertia of test particles plays no role in the global pattern of the geodesics.
>
> Inertia has to do with resistance when a force pushes a test particle off a timelike geodesic.
>
> Thats Higgs + QCD
> No gravity needed.
On Aug 21, 2011, at 11:19 PM,
Jack,
Responses below:
I don't understand then what you mean by "explanation" of the origin of inertia?
Yes, the question: What is the origin of inertia (and its measure mass)? can be interpreted in more than one way. The answer, or explanation, should encompass an explanation of why, when you push on an object, it pushes back on you with an equal and opposite force.
JS wrote: The usual explanation given by Eugene Wigner for that is from Noether's theorem applied to the T3 translation group. Every continuous symmetry Lie group of the action has a conserved "charge" quantity. T3 is generated by linear momentum "charge" elements of the Lie algebra.
In 1916 GR, i.e. T4 --> T4(x) + constraint of zero torsion
Tuv(matter source)^;v = 0
; is Levi-Civita connection
ensures local conservation of matter stress-energy current densities
Newton's 2nd Law on test particles is seemingly independent of that
D^2x^u/ds^2 = F^u(non-gravity force)/(rest mass)
I think Rovelli would say that the external force transfers momentum to the local gravity field piloting the test particle, and the local gravity field transfers equal and opposite momentum to the source of the external force. Agreed this is an interesting question. Here we must think of local stress-energy current densities since global field momenta integrals on spacelike surfaces need not be conserved in general.
For example, the universe is not symmetric under time translation, so the total universe energy is not conserved. The dark energy density is constant, hence the total dark energy is increasing as the universe's expansion accelerates - approaching a finite asymptote to be sure.
JW: That is, why is Newton's third law valid? There are, arguably, two parts to the answer to the question. One is: what is the stuff (Newton's "quantity of matter" updated) that has the property of inertia?
JS: Easy, the Higgs field + virtual gluon plasma inside the confined hadron "bags" of QCD (this also includes all virtual quanta including fermion-antifermion pairs, but the gluons are probably the dominant term).
JW: The second part is: why does it exert a reaction force on you, the agent providing the "external" force. Wilczek's argument using Einstein's second law and the usual calculation techniques for computing the local non-gravitational energy of a system is an answer to the first part of the full explanation of the origin of inertia. That is, it addresses (correctly) the question of 'what is the "quantity of matter"?' The answer being the sum of all of the non-gravitational energies in the region whose inertial mass is being computed. Wilczek, by the way, has been making this argument for a decade or more, and yet one still hears comments about the Higg's process being the origin of mass.
The answer to the second part of a complete explanation of the origin of inertia is only hinted at by Wilczek in his section "Owning up on mass" where he allows as how there is more to the issue of mass and inertia than simply calculating the local non-gravitational energy and dividing by c^2. There are several possible answers to the question: what causes the reaction force when you push on something. One is the Newtonian answer: because that is the nature of things that have inertia. Another is that there is some ambient field present that responds to the acceleration of local objects and produces the reaction force. I would add that the hypothetical ambient field is not the gravitational field to separate this answer from the third possibility. That third possibility is that inertial reaction forces are produced by the gravitational interaction (via the field) with all of the other "matter" [stuff that gravitates] in the universe. When this possibility is analyzed in some detail, it turns out that you have to adopt the Wheeler-Feynman [Hoyle-Narlikar] action-at-a-distance picture to get it to work correctly. The simple calculations of Sciama and Nordtvedt then show that the condition that must be met for gravity to produce inertial reaction forces is that the total scalar gravitational potential, phi, must be a locally measured invariant equal to the square of the speed of light, and so on . . . .
JS: Wheeler Feynman influence functional from the future gives "jerk" radiation resistance for sure. However, I don't think anyone takes the Sciama-Nordtvedt calculation as anything more that a primitive toy model. If it was the leading term in the real GR then it might explain your data as a first approximation. However, I do not understand what "phi" means unless you can express it in terms of Einstein's GR.
Do you mean
g00 = 1 + phi ?
But what guv are you using?
You can't use a cosmological metric to explain the rest mass of a single electron - the scales are too different, especially if Sakharov is correct and gravity is only an emergent "More is different" c-number low energy large scale effective field theory. So this issue of scales is very important.
The rest mass is key. Of course the kinetic component contributes. That is not the conceptual problem.
JW: No, rest mass in not key. Indeed, it is really almost irrelevant. This is the Higgs process as the origin of mass (the measure of inertia) argument. Rather than say the response again myself, I quote Wilczek:
JS interjected: While the precise numbers for the lepto-quarks may not be fundamental (e.g. cosmic landscape WAP), that they are not zero is essential for the QCD calculation to work. So I think you are mistaken there.
Wilzcek allegedly wrote :Then there's the Higgs particle, sometimes said to be the "origin of mass" or even "the God particle". . . . In brief the Higgs field (which is more fundamental than the particle) enables us to implement our vision of a universal cosmic superconductor and embodies the beautiful concept of spontaneous symmetry breaking. These ideas are deep, strange, glorious, and very probably true. But they don't explain the origin of mass -- let alone the origin of God. Although it's accurate to say that the Higgs field allows us to reconcile the existence of certain kinds of mass with the details of how the weak interactions work, that's a far cry from expaining the origin of mass or why the different masses have the values they do. And as we have seen, most of the mass of normal matter has an origin that has nothing whatsoever to do with Higgs particles."
JS wrote: This is a Red Herring. Wlizcek is only saying that MOST of the mass is in his QCD calculation. He certainly is not advocating a Machian origin of mass in the original 19th Century sense.
There is a very simple refutation of the whole idea.
Gravity is the pattern of geodesics.
JW: Well, no. Gravity is modeled by Einstein's field equations. And there is a lot more to it than just a pattern of geodesics.
JS: Wrong. Einstein's field equations simply determine the global pattern of geodesics. Tensor curvature is simply "geodesic deviation".
How much external non-gravity force is needed to produce a given tensor 4-acceleration on a test particle is irrelevant to Einstein's field equations.
The inertia of test particles plays no role in the global pattern of the geodesics.
JW: As test particles, of course not. The geodesics, by the way, usually computed are local, not global.
JS: Irrelevant - a quibble. A solution of Einstein's equations is implicitly the global geodesic pattern.
For example, in the SSS solution for static LNIFs
g00 = - 1/grr = 1 - rs/r
rs/r < 1
allows you to compute the global pattern of all null and timelike geodesics in the domain
rs < r < infinity
In Bohm's language the global pattern of geodesics is implicate in
Guv + kTuv = 0
(solving the field equations relative to a given class of detectors mind you)
explicates the geodesic pattern over a finite region of spacetime (i.e. "global")
the T4(x) GCTs + SO1,3 & tetrad transformations map the intrinsic geometry to different classes of locally coincident detectors
JW: For example, free fall in the vicinity of the Earth, or for planetary orbits, free fall in the vicinity of the Sun. The gravitational effect of distant matter in these cases is not explicitly taken into account. GRT, after all, is a local field theory.
JS: Inertia has to do with resistance when a force pushes a test particle off a timelike geodesic.
JW: Yes indeed. It is the thing that causes the equal and opposite reaction force that the agent forcing non-geodesic motion experiences. And the question is: what causes that reaction force?
JS: Thats Higgs + QCD
No gravity needed.
JW: Well, no. That's not right. As Wilczek points out [above], the Higgs process is irrelevant.
JS: No, as I showed above, you are misinterpreting Wilczek's test pulling it way out of context.
JW: QCD just allows you to calculate the gluon energy in the nucleons of the object being pushed. And even if you allow for Einstein's second law so you can divide by c^2 to get the mass, that still doesn't tell you what the cause of the inertial reaction force is.
JS: Basically it's Noether's theorem for the T3 group. You only get an inertial reaction force when the object in question is "clamped" (same as the hovering rockets firing in Hawking's picture below - there the steel of the cabin floor pushes you off geodesic with electrical force. You need to accelerate in order to stand still in curved spacetime. For example, our weight here on Earth. We are "clamped" to surface of Earth. We feel the electrical force of the Earth as weight because we are static LNIFs in the Earth's curvature field. Hawking explains this in "The Universe in a Nutshell."
JW: Mach's principle -- stated as gravity (due to chiefly distant matter [which is not included in the customary solutions of Einstein's equations for local geodesics]) being the cause of those local reaction forces, not stated as constraints on cosmological solutions -- provides the answer to this question. And it all fits. Mach's principle [defined as the gravitational origin of inertial reaction forces] is already implicit in the EEP with it's prohibition of localization of gravitational potential energy. And so on. . . .
Best,
Jim
On Aug 21, 2011, at 6:36 AM, "
Well Jack, respectfully I do not agree. Insisting that a "Machian" explanation of inertia should provide some independent means of calculating the energy of the binding gluons in nucleons is not reasonable. The method of calculating the local non-gravitational energy of an entity is not at issue. The QCD calculation of the energy of the binding gluons is no different from, for example, the electrodynamic calculation of the interaction energy of two electric charges brought into close proximity. That interaction energy, of course, provided by a bunch of virtual photons, contributes to the total local energy of the system, and contributes to the inertial mass of that system. If one says that something more than the simple calculation of that interaction energy is required to account for the origin of the mass the energy contributes to the system does not imply that some different method of calculating the energy is required.
The issue here is not the details of the calculation of the NON-gravittional energies of interactions of local sources and fields in QCD, QED, or classical electrodynamics. It is: does the calculation of those local energies of interaction divided by the square of the speed of light constitute a complete account of the origin of the inertial masses of the objects possessing those energies? If you read Wilczek's comments in "Owning up on mass", I think it fair to say that even he wouldn't claim that a calculation of the local non-gravitational energy of some entity constitutes a complete explanation of the origin of its mass. If it were, I should think he would have said that 100% of the origin of nucleon masses can be accounted for by multiplying the quark masses by c^2 and adding those energies to the gluon energy. He doesn't do that.
Putting the matter in terms of Einstein's second law, given that:
m = E / c^2,
is all one is required to do is calculate E to fully account for the origin of inertia and its measure mass? I assert that the answer to this question is "no". Why? Because no explanation has been provided for the origin of c^2 in the denominator -- the other half of the origin question. You can argue that this is just a consequence of SRT. But note that the dimension of c^2 is not just a velocity squared. It is also the dimension of gravitational potential -- and the universe we live in certainly has some non-vanishing gravitational potential. If you take Mach's principle as the assertion that inertial reaction forces are due to gravity, it follows that the total gravitational potential, phi, is a locally measured invariant like c and happens to be equal to c^2. So Einstein's second law says:
m = E / phi
and
m phi = E.
That is, the inertial mass of an entity with non-gravitational energy E is just that energy divided by the scalar gravitational potential -- because that energy is equal to the gravitational potential energy m phi. The origin of the inertial mass of the entity is the action of the gravitational field on the non-gravitational energy possessed by the entity.
So, calculating E is only half of explaining the origin of inertia (and its measure inertial mass). The other half is taking account of the action of gravity, which has nothing to do with the methods of calculating the local non-gravitational energies E.
I suppose you might ask the question: So what? Who cares if the origin of inertia is to be found in the gravitational interaction or not? Well, if you are looking for effects that might alter the inertia of, say, a starship (as you evidently are with your superconductor/metamaterial scheme), understanding how inertia arises would seem to be a desirable goal. Should either part of your scheme not work, some alternative will have to be found, or there won't be any starships and stargates. At any rate, not built by us. But it seems that there is an alternative. . . .
Best,
Jim
---------- Original Message ----------
From: Jack Sarfatti
Date: Sat, 20 Aug 2011 07:53:45 -0700
The point is that any alternative Machian explanation must at least be able to calculate what Wilczek did.
On Aug 20, 2011, at 7:35 AM, "
Gentlefolk,
Andrew has suggested that QCD + EP = origin of inertia. Unpacking Andrew's equation, QCD means the calculation carried out by Frank Wilczek, well at least the calculation described by Wilczek in his delightful book "The Lightness of Being" published several years ago, where he shows that more than 95% of the mass of the nucleons arises from the energy of the gluons that bind the quarks. For those of you who do not have (and have not read) Wilczek's book, I've excerpted the key passages therein in the attached word file. The first two and a half pages are devoted to Einstein's "second law". Then follow several pages where he talks about the QCD calculation of the proton mass. It is worth noting that in this section Wilczek allows that the quark restmasses and the quark-gluon coupling constant are treated as freely adjustable parameters in this calculation, which only returns the binding gluon energy. That is, these parameters are adjusted to get the right value of the gluon energy. This is perhaps a bit less than what one might hope for in an explanation of inertia. The last three pages are where Wilczek comes clean about the sense in which his argument can be taken as an "explanation" of inertia, and explicitly states what is not explained in his argument. The (adjustable) quark and (non-adjustable) lepton masses, for example.
In a nutshell, Wilczek's argument, which as far as it goes is correct, asserts that the inertial mass of nucleons is the sum of the non-gravitational energies associated with the bound quarks divided by the square of the speed of light. That is, Einstein's second law: m = E/c^2. This is more than 95% of the mass. Were one to multiply the (freely adjustable) quark masses assumed by c^2 and add that to the other energies, you'd have 100% of the inertial mass.
Now, the question is: If one adds the Equivalence Principle to Wilczek's QCD calculation, does this constitute a complete explanation of inertial mass? Well, if we are talking about the "weak" EP, the answer is arguably no. The WEP merely asserts that the passive gravitational mass and the inertial mass are the same (and drop out of F = ma = GmM/r^2 => a = GM/r^2). Why? Because the active gravitational mass is left unaddressed, and in any event, it is the Einstein EP that is required to get GRT. The EEP differs from the WEP in that the EEP forbids the localization of gravitational potential energies. This may seem an incidental feature of the EEP unrelated to the issue of the origin of inertia and its measure, mass. But it is crucial. Active gravitational mass gets included in the EP in the variant known as the Strong EP (SEP).
Now, as I have already pointed out in those longer missives on Mach's principle and the origin of inertia (which you may not have read carefully owing to their length and tortuous attention to details), the EEP already contains implicitly Mach's principle as I define it. How? It's the prohibition of the inclusion of localizable gravitational potential energies that's related to Mach's principle. [By the way, I also attach a very nice 2004 Physics Today article by Wilczek on Mach's principle for you diversion.]
Recall, I do not define Mach's principle in the customary way by talking about relational motion and cosmology and all that. My definition of Mach's principle is the one that is restricted to its local physical content: inertial reaction forces are caused by the gravitational action of the rest of the "matter" in the causally connected universe. This assertion is true provided that the total scalar gravitational potential is just equal to the square of the speed of light. (I have also attached the few pages from Sciama and Nordtvedt where this conclusion about phi and c^2 can be inferred.) In order that this condition be true everywhere and everywhen, phi must be a locally measured invariant like c. Two things follow immediately from this inference. One, gravitational potential energy has the property required by the EEP, and as a result inertial reaction forces of gravitational origin equal applied forces universally. And two, E = mc^2 = m*phi. Or, m = E/c^2 = E/phi, which is just Einstein's second law with E non-gravitational energy. Now you have an "explanation" of inertia. It is just the action of gravity on non-gravitational energy. And you also have an explanation of the origin of inertial reaction forces. And it is simple enough for me to write this out without having to use an equation editor. :-)
I also note that Mach's principle requires an action-at-a-distance representation of gravity (as Hoyle and Narlikar argued many years ago and Jack now includes in his future hologram view). Either that, or the "constraint" approach of Lynden-Bell and (independently) Wheeler.
On other matters, this past week saw attention drawn to matters related to the "polarizable vacuum", a topic of particular interest to Hal, Eric, and Sonny (among others). I attach Hal's 2006 FoP paper on the subject as you may find it of interest.
Jack circulated to another list an abstract of the paper he will be presenting at the 100 Year Starship convention in Orlando next month. It's his superconductor/metamaterial scheme which has been placed in the "exotic propulsion" section. The link to the agenda for that meeting is . An interesting collection of presentations. And some lucky organization will get a half megabuck (and a kiss goodbye) from DARPA and NASA on 11/11/11 with the proviso that the organization commit to working on starships for 100 years.
On the experimental front, progress is slow. Bruce's dual resonance matcher didn't work as well as hoped on my last LA trip. And I didn't have time to track the source of the problem (thanks to the USPS, which had thoroughly loused up Carole's and my forwarding, necessitating a trip to the local PO). One of the new stacks, however, has been assembled into the mounting hardware, and I hope to get it onto the balance and do some preliminary testing next trip (next week). Comparison of the electrical characteristics of the new stack with the old promises that the new stack should perform nicely, with a little luck.
May you have a good weekend,
Jim
---------- Original Message ----------
From: "
Subject: Re: QCD + EP = origin of inertia
Date: Thu, 18 Aug 2011 03:31:48 GMT
Hi Bernie/all,
I'm responding to this particular email because it is the most recent in this thread, not because I am singling out anyone's response.
The conversation, from my point of view, has been interesting as it sheds light for me on how others are looking at the issues surrounding the "origin of mass" and Mach's principle. As it turns out, getting this right is central to understanding "Mach effects", and from the conversation it is clear to me now that I haven't succeeded in putting this in terms that are easy to follow. So, I'm going to take another pass at this, and I'm going to include in that pass a few excerpts from original papers so that there can be no confusion over what those authors say, and what I say that they say.
I've been traveling the past few days with only intermitent access to email, so it will be tomorrow or the next day before I can get this done. But it will get done. :-)
Maybe we can make a little progress. . . .
My very best,
Jim
---------- Original Message ----------
From: Bernard Haisch To: "Andrew"
Date: Wed, 17 Aug 2011 12:56:42 -0700
I believe the inertial force can be traced to Doppler shifts of the photons inside the sphere.
Bernie
Jim,
1916 typo, but yes. An internally-silvered sphere containing photons and/or fields will accelerate more slowly than an empty sphere under the influence of the same externally applied force. Therefore Higgs does not account 100% for inertia, as you say.
Andrew
-- <<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<<>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>>
Dr. Bernard Haisch
